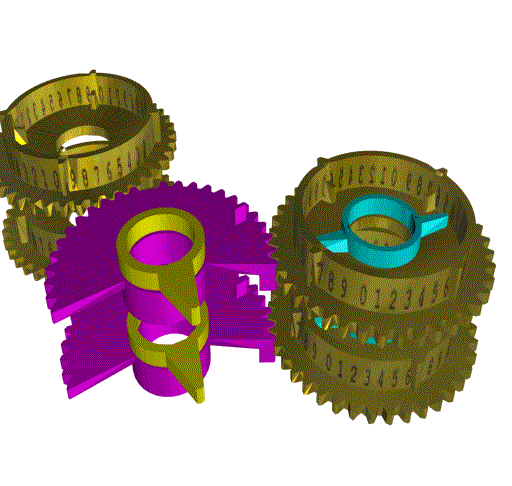
The image shows the sector wheels already in the down position, engaged with the figure wheels on the odd and even axles.
| < Previous | Up ^ | Next > |
In the following animations, the different parts have been color-coded to make it simple to refer them. Only the parts relevant to each stage are shown.
The whole process of calculating a new value is divided in two half cycles. As mentioned before, there are eight axles carrying each a full 31 digit number. They are divided in two groups, even and odd, which alternate in their movements. The figure wheels to the right are just two digits of an odd axle, the ones in the left are on an even axle. The animations show just one half cycle, in this case, the addition of the value on the odd axle to the value already on the even axle. What is shown in the animation, also happens simultaneously in four other axles, and will happen on the remaining four just half a cycle afterward.
The animations are quite large so they have been rendered in 160*120 pixels, so they remain under 250kbytes. A larger, static image has been included to help appreciate the detail, though in file size is actually small.
 |
The bronze figure wheels on the right are on an odd axle. The wheel
below is the least significant digit. As shown they hold the number 35. The
wheels in the background, at left, are on an even axle and they are showing a 48.
The half gears in magenta are called sector wheels. Both kinds of wheels have
restore arms, the ones for the figure wheels are shown in light blue, the ones for the
sector wheels in yellow. The restore arm for the bottom figure wheel is barely visible
through the gap in between the figure wheels. The even figure wheels, in the background,
to the left, also have restore arms, but they are not shown since they are idle in this
half cycle. Likewise, there are sector wheels linking the even figure wheels in the back
to the odd figure wheels further away. The image shows the sector wheels already in the down position, engaged with the figure wheels on the odd and even axles. |

The animation above shows the process of adding a digit. Though the image loops forever, it is easy to notice the start of the cycle because there is a sudden jump in the animation.
The process starts with the half gears in magenta, the sector wheels, moving down and engaging the figure wheels. The figure wheel restore arms also goes down along the sector wheels. Once they both get to their lower position, the restore arms start to turn.
At some point, the restore arms will hit two of the inner teeth of the figure wheel, and will carry it along. Notice in the animation that since the two figure wheels are at different positions, that is, representing different numbers, they start moving at different points in time.
The restore arm always moves the figure wheels back to zero. In doing so, whatever value they carried is transferred via the sector wheels to the receiving figure wheels, shown at the back. The restore arms come down at the position that the wheel would occupy should it be holding a 9. If the figure wheel had a nine, the restore arm would engage it right away and carry it over 9 units, transferring those to the sector wheel and the next figure wheel. The lower the number, the longer it takes for the restore arm to engage the inner tooth of the figure wheel, so there is less left to transfer to the sector wheel.
At the end of this process, the figure wheels in the background hold the addition, as we expected while the figure wheels in the foreground hold zero. This is not good, since we still need these wheels to hold their previous value so it can be used in the next cycle. That is where the sector wheel restore arm comes into play. After all, the sector wheels also got a copy of whatever value was held by the foreground figure wheels.
| < Previous | Up ^ | Next > |